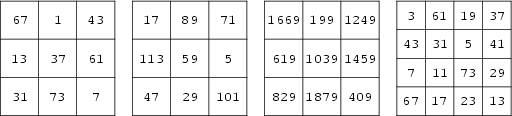
A prime magic square is a magic square consisting only of prime numbers (although the number 1 is sometimes allowed in such squares). The left square is the prime magic square (containing a 1) having the smallest possible magic constant, and was discovered by Dudeney in 1917 (Dudeney 1970; Gardner 1984, p. 86). The second square is the magic square consisting of primes only having the smallest possible magic constant (Madachy 1979, p. 95; attributed to R. Ondrejka). The third square is the prime magic square consisting of consecutive primes having the smallest possible magic constant (Madachy 1979, p. 95; attributed to R. Ondrejka). The prime magic square on the right was found by A. W. Johnson, Jr. (Dewdney 1988).

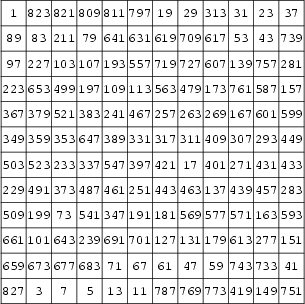
According to a 1913 proof of J. N. Muncey (cited in Gardner 1984, pp. 86-87), the smallest magic square composed of consecutive odd primes including the number 1 is of order 12, illustrated above.

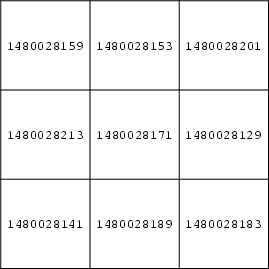
The square whose entries are consecutive primes illustrated above was discovered by Nelson (Guy 1994, p. 18; Rivera) in response to a challenge by Martin Gardner. Nelson collected Gardner's $100 prize, and also found 20 other such squares (Guy 1994, p. 18).

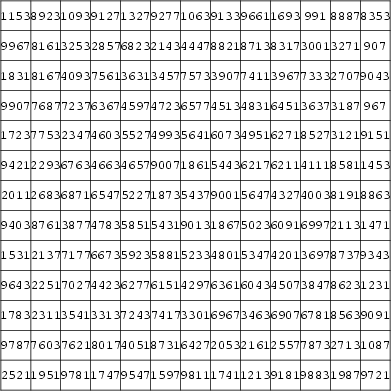
The amazing square above (Madachy 1979, pp. 93-94) is a prime magic border square, so that the , , ..., and subsquares are all also prime magic squares.

Magic Square, Prime Array, Prime Number