Let G be a subgroup of the modular group gamma. Then an open subset ![]() of the upper half-plane H is called a fundamental region of G if
of the upper half-plane H is called a fundamental region of G if
- 1. No two distinct points of
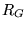 are equivalent under G,
are equivalent under G, - 2. If
 ,
,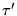 in the closure of
in the closure of 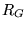 such that
such that 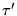 is equivalent to
is equivalent to 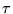 under G.
under G.
A fundamental region ![]() of the modular group gamma is given by
of the modular group gamma is given by ![]() such that
such that ![]() and
and ![]() ,
,![]() is the complex conjugate of
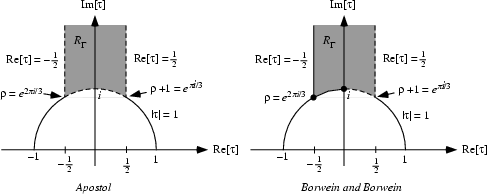
is the complex conjugate of ![]() (Apostol 1997, p. 31). Borwein and Borwein (1987, p. 113) define the boundaries of the region slightly differently by including the boundary points with
(Apostol 1997, p. 31). Borwein and Borwein (1987, p. 113) define the boundaries of the region slightly differently by including the boundary points with ![]() .
.
![]()
Modular Group Gamma, Modular Group Lambda, Upper Half-Plane, Valence
![]()
![]()
Apostol, T. M. "Fundamental Region." §2.3 in Modular Functions and Dirichlet Series in Number Theory, 2nd ed. New York: Springer-Verlag, pp. 30-34, 1997.
Borwein, J. M. and Borwein, P. B. Pi & the AGM: A Study in Analytic Number Theory and Computational Complexity. New York: Wiley, pp. 112-113, 1987.
![]()